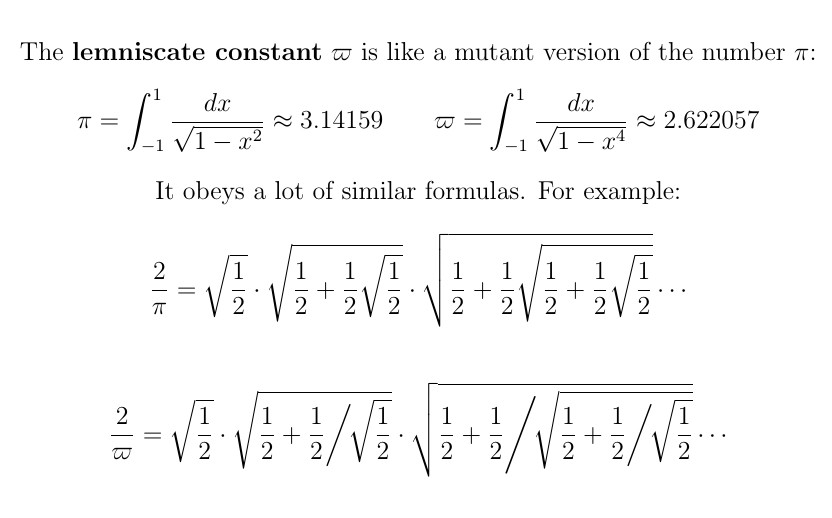
The number pi has an evil twin! It's a number called ϖ with many properties similar to π. There are even mutant trig functions connected to this number, called sl and cl.
So maybe while you were studying trig in high school, some kid in another galaxy was having to memorize all the identities for these other functions.
I doubt it. Just as pi and trig functions are connected to the circle, this number ϖ and its mutant trig functions are connected to a curve shaped like the symbol for infinity, ∞. But this curve is just less important than the circle. I'm not enough of a cultural relativist to believe there's a civilization that cares more about the shape ∞ than the shape ◯.
This ∞-shaped curve is called a 'leminscate', and ϖ is called the 'lemniscate constant'. I'll show you the leminiscate in my next post.
A civilization will probably only get interested in ϖ when it gets interested in the lemniscate.... or the deeper math it's connected to. On our planet, it was Bernoulli, Euler and Gauss who discovered this math.
(Why does unicode even have the symbol ϖ? Here's why: it's a script version of the Greek letter pi, sometimes called 'varpi' or 'pomega'.)
(1/n)
Take 2 points. Draw all the curves where the product of the distances from these 2 points is some constant or other. These are called the 'ovals of Cassini'.
There's one that's special, shaped like ∞. This is the 'lemniscate'. This is the one connected to pi's evil twin.
Here's a formula for the lemniscate in polar coordinates:
r² = cos2θ
Just as the perimeter of a circle is 2π, the perimeter of this curve is 2ϖ where
ϖ ≈ 2.62205755...
People have computed this number to over a trillion digits.
Just as we can use the circle to define the trig functions sin and cos, we can use this curve to define functions called sl and cl. Most of the usual trig identities have mutant versions that work for sl and cl. For example just as we have
sin²θ + cos²θ = 1
we have
sl²θ + cl²θ + sl²θ cl²θ = 1
To see a graph of sl and cl, go here:
https://en.wikipedia.org/wiki/Lemniscate_elliptic_functions
But I want to show you some formulas for π and ϖ!
(2/n)
Lots of nice formulas for π have partners for the number ϖ!
Back before Twitter became a Nazi bar, I issued a challenge there: find a whole series of numbers like pi, each with its own bunch of formulas. @duetosymmetry took me up on this and invented the numbers ϖₙ:
https://math.ucr.edu/home/baez//diary/june_2022.html#june_12
π is ϖ₂ and ϖ is ϖ₄. So between them there's another interesting mutant version of pi!
(3/n)
If you're a serious mathematician who has been suffering through all this pop math fluff, wondering what's *really* going on here, let me finally come out and say:
The reason ϖ and its mutant trig functions are important is that they're connected to one of the most symmetrical elliptic curves of all, the Gaussian elliptic curve!
You can get this by taking the complex plane and modding out by a square lattice. Any lattice in the complex plane gives an elliptic curve and elliptic functions. But this particular case is especially nice, because a square is more symmetrical than other parallelograms, so it was discovered early.
Gauss discovered that this elliptic curve is connected to the 'arithmetic-geometric mean', defined below. And the arithmetic-geometric mean of 1 and √2 is the ratio π/ϖ. This number is called Gauss' constant.
https://en.wikipedia.org/wiki/Lemniscate_constant
I believe the higher numbers ϖₙ are similarly related to certain specially nice hyperelliptic functions. Hyperelliptic functions come from hyperelliptic curves, which are defined to be branched double covers of the Riemann sphere. I think the numbers ϖₙ are connected to some very symmetrical hyperelliptic curves, but I haven't checked.
Happy holidays!
(4/n, n = 4)